Average Gain obtained by averaging -(Linear Loss ) scenarios, i.e., it is a linear function with coefficients obtained by averaging  coefficients of  -(Linear Loss) scenarios.

 

Syntax

 

Parameters

matrix        is a Matrix of Scenarios:

       

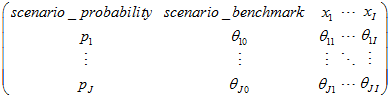
where the header row contains names of variables (except scenario_probability, and scenario_benchmark). Other rows contain numerical data. The scenario_probability, and scenario_benchmark columns are optional.

 

Mathematical Definition

Average Gain function is calculated as follows

,

where:

E denotes the expectation sign;

is Average Loss function,

random vector has components and J vector scenarios, ,

random value , which is the i-th component of the random vector, ,  has J discrete scenarios ,

is probability of the scenario ,

is Loss Function (See section Loss and Gain Functions),

is an argument of Average Gain function.

 

Example

 

Case Studies with Average Gain

 

See also

Average Loss, Average Max, Average Max for Gain, Average Max Deviations, Average Max Deviation for Gain